pmarc
Mechanical
- Sep 2, 2008
- 3,227
Hello,
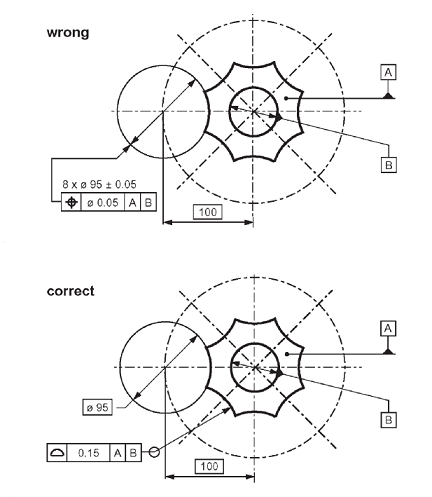
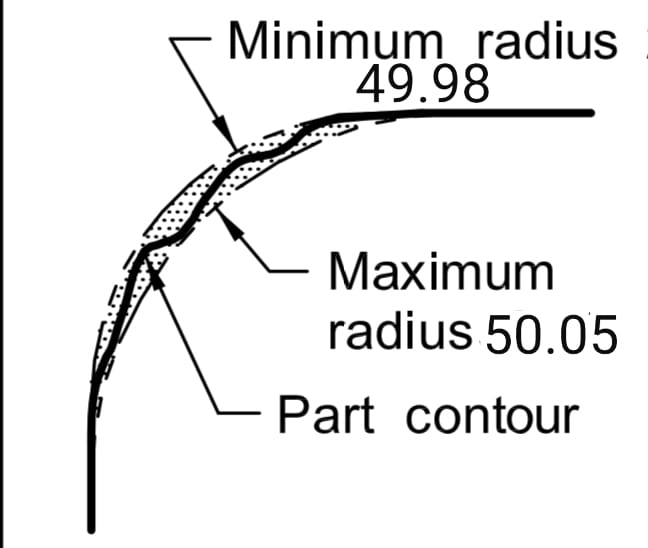
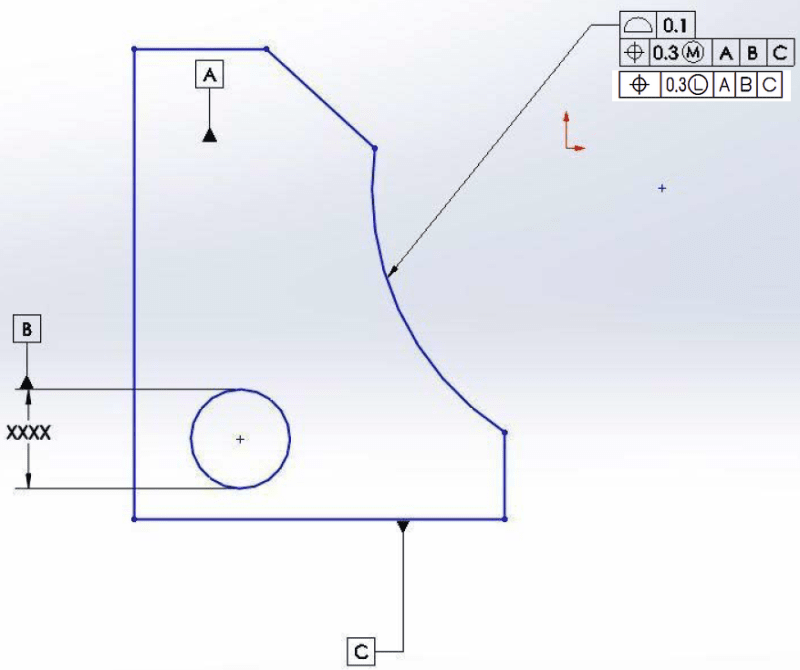
Below is a snapshot taken from the most recent Tec-Ease tip available on their website. My question would be following: Does anyone think that the true profile between points S and T (clockwise) has not been fully defined? I am specifically thinking about close vicinity of points S and T. Thank you!

Below is a snapshot taken from the most recent Tec-Ease tip available on their website. My question would be following: Does anyone think that the true profile between points S and T (clockwise) has not been fully defined? I am specifically thinking about close vicinity of points S and T. Thank you!


![[wink] [wink] [wink]](/data/assets/smilies/wink.gif) :
:
![[smile] [smile] [smile]](/data/assets/smilies/smile.gif) .
.![[ponder] [ponder] [ponder]](/data/assets/smilies/ponder.gif)