Is this real? 3

[Enhineyero]

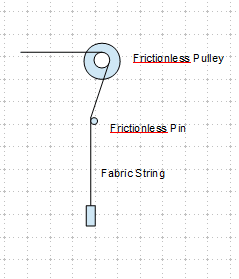
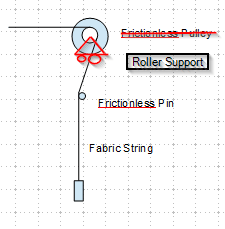
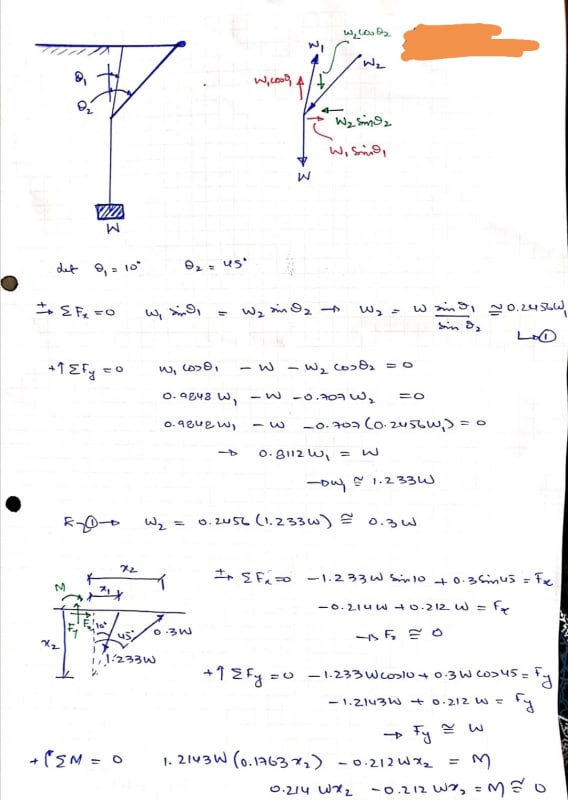
Found this video on the internet showing a cantilevered system without a counterweight. I don't think it is real!

https://bilimkocu.com/?p=1952

also on youtube:

https://youtu.be/CsmjjOMQg34

Let me know your thoughts....

 

[SnTMan]

Well, I can't find it anywhere but I am sure this was discussed in the past as the Devil's Hook or some such...

Regards,

Mike

The problem with sloppy work is that the supply FAR EXCEEDS the demand

 

[Retrograde]

There is no need for the horizontal friction at the top for this to be stable.

 

[IRstuff]

@Megastructures
The issue with inserting images in an edited post is a known problem.

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert!

https://www.youtube.com/watch?v=BKorP55Aqvg

faq731-376 forum1529 Entire Forum list

http://www.eng-tips.com/forumlist.cfm

 

[r13]

The friction is along the string on contact surface, the match provides pivot point.

 

[IRstuff]

Friction is absolutely not necessary; the Devil's Hook cited above uses no friction. If you carefully look at the belt, its profile isn't horrifically different than that of the cord in the video.

Likewise, Megastucture's figures require zero friction; stability is guaranteed by moving the CG inboard of the pivot point on the edge.

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert!

https://www.youtube.com/watch?v=BKorP55Aqvg

faq731-376 forum1529 Entire Forum list

http://www.eng-tips.com/forumlist.cfm

 

[r13]

Friction is absolutely not necessary;

I wouldn't be so sure. Who can write equilibrium equations for this system, say the hanging weight >= weight of string?

 

[MegaStructures]

That's not the same mechanism @retired13. The compression strut is required to create a force couple. Also, the load under the table is resisted vertically by contact between the match and table. In your figure you provide no vertical, or lateral, restraint, which of course creates a system that cannot be in equilibrium.

“Any idiot can build a bridge that stands, but it takes an engineer to build a bridge that barely stands.”

 

[MegaStructures]

“Any idiot can build a bridge that stands, but it takes an engineer to build a bridge that barely stands.”

 

[r13]

Mega,

Very good. How about this now?

 

[Retrograde]

So what's supporting the pulley and the pin? This is a completely different mechanism than what is being discussed.

 

[r13]

Assume the pulley and pin have out of page supports that can take vertical load. The concept is the same.

 

[Retrograde]

The concept is not remotely the same!

In the video, the match at the top is taking the load through shear. Your sketch has a fabric string that will not be able to take shear.

 

[r13]

You didn't think hard enough

 

[Compositepro]

Friction and compression are all that hold joints at matches and string together. The free body diagrams are simple.

 

[IRstuff]

@retired13
Your drawing bears zero resemblance to the problem described, since there is no W2 anywhere in the problem, other than the weight that held the match in place, initially. By your drawing, removal of the weight would immediately result in everything falling, and there cannot possibly enough friction to keep the string (match) from sliding off the table. You are solving some other problem of your own creation.

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert!

https://www.youtube.com/watch?v=BKorP55Aqvg

faq731-376 forum1529 Entire Forum list

http://www.eng-tips.com/forumlist.cfm

 

[r13]

IR,

See Composite' response. I am amused that a chemical engineer knows structural tricks.

 

[IRstuff]

Compositepro's description only applies to the 4 joints created by the addition two matches and not to the original match.

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert!

https://www.youtube.com/watch?v=BKorP55Aqvg

faq731-376 forum1529 Entire Forum list

http://www.eng-tips.com/forumlist.cfm

 

[human909]

I can't believe this thread is still going. Isn't this something we played around with as kids? Alternatively if you are still confused it isn't too hard to do it yourself.

 

[Enhineyero]

I can't believe this thread is still going. Isn't this something we played around with as kids? Alternatively if you are still confused it isn't too hard to do it yourself.

Shows why many of us chose engineering. Or did it choose us?

 

[Blackstar123]

It isn't difficult to prove that the problem is statically stable.. if my calculations are right, zero (or nearly close to zero) horizontal reaction and overturning moment is produced at the support.