Enhineyero
Structural
- Sep 1, 2011
- 285
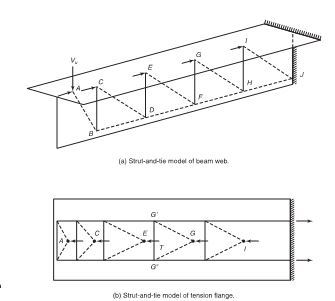
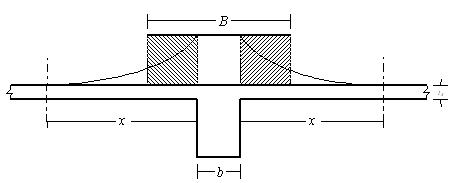
I was wondering, why is the T-beam effective width a function of the span length? or to be more precise for relevant codes a function of the points of contraflexure for sagging bending moments.
Hope someone could help
Hope someone could help